BÀI TẬP TUẦN 2
– Liên hệ giữa phép nhân (phép chia ) và phép khai phương
– 1 số hệ thức cạnh và đường cao trong tam giác vuông
Bài 1: Tính
a) displaystylesqrt90.360
b) displaystylesqrt52.sqrt13
c) displaystylesqrtfrac652−522196
d) displaystylefracsqrt13sqrt208
Bài 2: Thực hiện phép tính
a) displaystylesqrt1,6.sqrt250+sqrt19,6:sqrt4,9
b)displaystyleleft(sqrtfrac23+sqrtfrac503−sqrt24right).sqrt6
c) displaystyleleft(sqrtfrac13−sqrtfrac43+sqrt3right):sqrt3
d) displaystylesqrt3+sqrt5.sqrt2
Bài 3: Rút gọn biểu thức:
a) displaystylefracsqrt10−sqrt15sqrt8−sqrt12
b) displaystylefrac5+sqrt5sqrt10+sqrt2
c) displaystylefracxsqrty+ysqrtxx+2sqrtxy+y( với x ≥ 0, y ≥ 0, xy ≠ 0
d) displaystylefracxsqrtx+ysqrtyx−sqrtxy+y
Bài 4: Rút gọn biểu thức sau:
a) A = displaystylesqrt8+2sqrt15−sqrt8−2sqrt15
b) B = displaystylesqrt3−sqrt5−sqrt3+sqrt5( 2 cách)
c*) C =displaystylesqrt2+sqrt3.sqrt2+sqrt2+sqrt3.sqrt2+sqrt2+sqrt2+sqrt3.sqrt2−sqrt2+sqrt2+sqrt3
d) displaystylefracsqrt15−sqrt5sqrt3−1+frac5−2sqrt52sqrt5−4
e) displaystylefrac3+2sqrt3sqrt3+frac2+sqrt2sqrt2+1−(sqrt2+sqrt3)
Bài 5: Tìm x, biết:
a) displaystylesqrtx−5+sqrt4x−20−frac15sqrt9x−45=3
b) displaystyle2sqrt9x−27−frac15sqrt25x−75−frac17sqrt49x−147=20
c) displaystylesqrtx2−2x+9=2x−3
d)displaystylesqrt6−2x2+1=x
e) displaystylesqrtx−3−2sqrtx2−9=0
f) displaystylefracsqrtx−3sqrt2x+1=2
Bài 6*: Tìm giá trị lớn nhất của biểu thức
a) A = displaystylefracsqrtx2+x+3sqrtx2+x+1
b) B = displaystylesqrt1−x.sqrtx+3
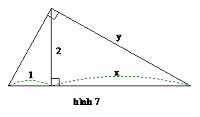
Bài 7: Tìm x, y có trên hình vẽ sau :

Bài 9*: Cho tam giác ABC cân tại A, đường cao AH và BK. Chứng minh:
displaystylefrac1BK2=frac1BC2+frac14AH2
