BÀI TẬP TUẦN 1
– Căn thức bậc hai displaystylesqrtA2=left|Aright|
– 1 số hệ thức cạnh và đường cao trong tam giác vuông
Bài 1: So sánh
a) 5 và displaystylesqrt26
b) displaystyle−sqrt26 và displaystyle−sqrt29
c) displaystylesqrt37+sqrt10 và displaystylesqrt80
Bài 2: Tính giá trị biểu thức:
a) displaystylesqrtfrac2549−sqrtfrac116+sqrt(−2)2
b) displaystyleleft(sqrt3right)2sqrt0,25−0,2sqrtleft(−3right)2
c) displaystylesqrt1132−1122
d) displaystylesqrtleft(−0,1right)4+sqrt582−422
e) displaystylesqrtleft(sqrt10−3right)2+sqrtleft(sqrt10−4right)2
f) displaystylesqrt11+6sqrt2+sqrt11−6sqrt2
g)displaystylesqrt29+12sqrt5+sqrt29−12sqrt5
Bài 3: Tìm x để biểu thức sau có nghĩa
a) displaystylesqrt3x−1
b) displaystylesqrtfrac2x−1
c) displaystylesqrt5+x2
d)displaystylesqrtfracx−12x−4
Bài 4: Tìm x, biết:
a) displaystylesqrtx2=left|−7right|
b) displaystylefracsqrt9−4sqrt52−sqrt5displaystylesqrt36x2=10
c) displaystylesqrt4x2−4x+1=5
d) displaystylesqrtx2−4x+25=5
e) displaystylesqrtx<2
f) displaystylefrac4−2sqrtxsqrtx+1>0
g*) displaystylesqrtx+4sqrtx−4=2
h*) displaystylesqrt2x−2+2sqrt2x−3+sqrt2x+13+8sqrt2x−3=5
Bài 5: Phân tích đa thức thành nhân tử, rút gọn biểu thức:
a) x2 – 10
b) displaystylex−4sqrtx+4
c) displaystylefracx−6sqrtx+9x−9
d) displaystylefracsqrt9−4sqrt52−sqrt5
e*) displaystylefracsqrt9+2sqrt2+2sqrt3+2sqrt2sqrt36+3sqrt2+3sqrt3
Bài 6*: Tìm x,y,z biết: displaystylefracx+y+z2−3020=sqrtx−2017+2sqrty−2018+3sqrtz−2019
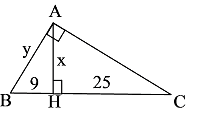
Bài 7: Tìm x, y có trên hình vẽ sau :
Bài 8: Cho tam giác ABC, đường cao AH, biết AB2 = BH.BC. Chứng minh tam giác ABC vuông.
Bài 9: Cho ΔABC vuông tại A, đường cao AH. Biết HB = 3,6cm ; HC = 6,4cm
a)Tính độ dài các đoạn thẳng: AB, AC, AH.
b) Kẻ HEdisplaystylebotAB ; HFdisplaystylebotAC. Chứng minh rằng: AB.AE = AC.AF
Bài 10: Cho tam giác ABC vuông ở A, phân giác AD, đường cao AH. Biết CD = 68cm, BD = 51cm. Tính BH, HC.
Bài 11: Cho hình thang ABCD có displaystylewidehatBtext=widehattextCtext=9text0texto, hai đường chéo vuông góc với nhau tại H. Biết rằng AB = displaystyle3sqrt5cm; HA = 3cm. Chứng minh rằng:
a) HA : HB : HC : HD = 1 : 2 : 4 : 8
b) displaystylefractext1textAtextBtext2−fractext1textCtextDtext2=fractext1textHtextBtext2−fractext1textHtextCtext2
